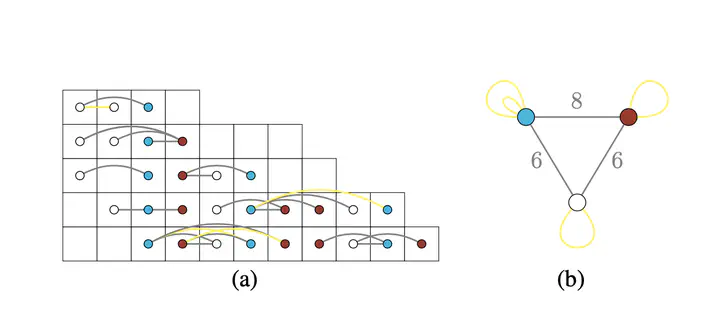
Abstract
We prove a combinatorial formula for Macdonald cumulants which generalizes the celebrated formula of Haglund, Haiman and Loehr for Macdonald polynomials. We provide several applications of our formula. Firstly, it allows us to give a new, constructive proof of a strong factorization property of Macdonald polynomials proven recently by the author of this paper. Moreover, we prove that Macdonald cumulants are $q,t$-positive in the monomial and in the fundamental quasisymmetric bases. Furthermore, we use our formula to prove the recent higher-order Macdonald positivity conjecture for the coefficients of the Schur polynomials indexed by hooks. Our combinatorial formula relates Macdonald cumulants to the generating function of $G$-parking functions, or equivalently to a certain specialization of the Tutte polynomials.